Gradient
 From Conservapedia - Reading time: 2 min
From Conservapedia - Reading time: 2 min

|
This article/section deals with mathematical concepts appropriate for late high school or early college. |
In mathematics, the gradient is a vector associated to a point  of a differentiable function
of a differentiable function  which takes real values. Specifically, the gradient at
which takes real values. Specifically, the gradient at  is a vector in
is a vector in  which points in the direction in which
which points in the direction in which  increases most rapidly at
increases most rapidly at  . The magnitude of the gradient at
. The magnitude of the gradient at  is equal to the maximum directional derivative of
is equal to the maximum directional derivative of  at
at  . The gradient is an extension of the idea of derivative to functions with more than one variable.
. The gradient is an extension of the idea of derivative to functions with more than one variable.
Stated another way, a gradient is a vector that has coordinate components that consist of the partial derivatives of a function with respect to each of its variables. For example, if  , then
, then
 .
.
Observe that in this case, the gradient vector  is orthogonal to the "level curve" defined by
is orthogonal to the "level curve" defined by  , which here is a circle: the gradient points outward from the origin, which is the direction of steepest increase of
, which here is a circle: the gradient points outward from the origin, which is the direction of steepest increase of  , and vectors outward from the origin are perpendicular to circles centered at the origin. We'll see later that this is a case of a more general property of the gradient.
, and vectors outward from the origin are perpendicular to circles centered at the origin. We'll see later that this is a case of a more general property of the gradient.
More precisely, we define the gradient,  of
of  to be the vector field:
to be the vector field:

consisting of the various partial derivatives of  . If
. If  is a unit vector in
is a unit vector in  , then, by the chain rule, the directional derivative of
, then, by the chain rule, the directional derivative of  in the direction of
in the direction of  is simply the dot product:
is simply the dot product:

Evidently by the Cauchy-Schwartz inequality, the directional derivative in the direction  is maximal in the direction of the gradient, and equal to
is maximal in the direction of the gradient, and equal to  for
for  a unit vector in the direction of the gradient.
a unit vector in the direction of the gradient.
Properties of the Gradient[edit]
If  is a differentiable function with smooth level sets
is a differentiable function with smooth level sets  , then the gradient vector field
, then the gradient vector field  is perpendicular to the level sets of
is perpendicular to the level sets of  . For fix a level set
. For fix a level set  , and let
, and let  be a vector tangent to
be a vector tangent to 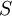 at
at  . Then we can find a curve
. Then we can find a curve  on
on 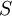 with
with  . Now
. Now

since 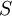 is a level set. Taking derivatives of both sides and applying the chain rule, we get that
is a level set. Taking derivatives of both sides and applying the chain rule, we get that

Thus,  is perpendicular to
is perpendicular to  at
at  , i.e., the gradient of
, i.e., the gradient of  is perpendicular to the level sets of
is perpendicular to the level sets of  .
.
 KSF
KSF