Lorentz transformation
 From Conservapedia - Reading time: 1 min
From Conservapedia - Reading time: 1 min
Lorentz transformations form the group of linear isometries of Minkowski space.
In physics, a Lorentz transformation is the conversion of space and time between two different inertial frames of reference.
According to Einstein the principles of Special Relativity are mathematically expressed by Lorentz transformations owing to which it is possible to transform equations for mechanical and electromechanical phenomena between inertial systems.[1]
Contents
Mathematics of the Transforms[edit]
Displacement and Time[edit]
The transformation from one coordinate system  to another system,
to another system,  , moving past this one at speed u, and with
, moving past this one at speed u, and with  and
and  axes colinear is:
axes colinear is:


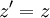

where  is the Lorentz factor[2].
is the Lorentz factor[2].
Velocity Transforms[edit]
The velocity transforms can be found by differentiating the displacement transforms with respect to time. For the above coordinate systems, we find:


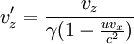
Note how the transformations for velocity in the y and z directions also depend on the velocity of the particle in the x direction, not just the relative speed between the frames of reference. This is different to the displacement transformations, in which y and z are independent of x.
See also[edit]
References[edit]
- ↑ Michal Andrle. Whitheadova Filosofie Přírody (in Czech). Prague: Charles University, 155. ISBN 978-80-87378-22-9.
- ↑ Bradley W. Carroll, Dale A Ostlie. An Introduction to Modern Astrophysics (in English). London: Pearson.
 KSF
KSF