Barrier
From Encyclopedia of Mathematics - Reading time: 2 min
Lebesgue barrier, in potential theory
A function the existence of which is a necessary and sufficient condition for the regularity of a boundary point with respect to the behaviour of a generalized solution of the Dirichlet problem at that point (cf. Perron method; Regular boundary point).
Let 
be a domain in a Euclidean space 
, 
, and let 
be a point on its boundary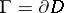 
. A barrier for the point 
is any function 
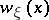, continuous in the intersection 
of the closed domain 
with some ball 
with centre at 
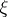, which is superharmonic in 
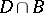and positive in 
, except at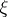 
, at which it vanishes. For instance, if 
and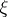 
is any boundary point for which there exists a closed ball 
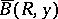in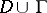 
which meets 
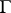only in 
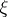, one can take as a barrier the harmonic function
 |
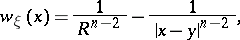where 
is the radius of 
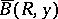and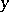 
is its centre.
A barrier in the theory of functions of (several) complex variables is a function the existence of which for all boundary points of the domain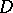 
implies that 
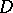is a domain of holomorphy. Let 
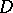be a domain in the complex space 
, 
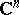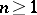, and let 
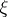be a point of the boundary 
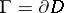. Any analytic function 
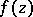in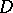 
with a singular point at 
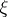will then be a barrier at 
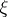. Thus, the function 
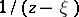is a barrier for the boundary point 
of any plane domain 
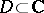. There also exists a barrier at any point 
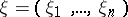of the boundary of the ball
 |
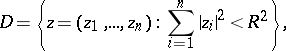e.g. the function 
.
A barrier exists at a boundary point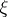 
of a domain 
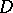if there is an analytic function defined in 
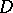that is unbounded at 
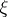, i.e. is such that for some sequence of points 
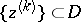which converges to 
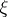one has:
 |
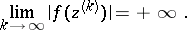The converse is true for domains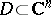 
in the following strong form: For any set 
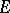of boundary points of a domain 
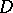at which a barrier exists, one can find a function holomorphic in 
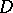which is unbounded at all points of 
. If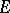 
is everywhere dense in the boundary of 
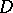, then 
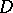is a domain of holomorphy.
References[edit]
| [1] | R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German) MR0195654 |
| [2] | V.S. Vladimirov, "Methods of the theory of functions of several complex variables" , M.I.T. (1966) pp. Chapt. 3 (Translated from Russian) |
| [3] | B.V. Shabat, "Introduction of complex analysis" , 1–2 , Moscow (1985) pp. Chapt. 3 (In Russian) Zbl 0578.32001 Zbl 0574.30001 |
Comments[edit]
Good English references for the Lebesgue barrier are [a1] and [a2].
References[edit]
| [a1] | W.K. Hayman, P.B. Kennedy, "Subharmonic functions" , 1 , Cambridge Univ. Press (1976) MR0460672 MR0419791 MR0412442 MR0442324 Zbl 0419.31001 Zbl 0339.31003 Zbl 0328.33011 |
| [a2] | L.L. Helms, "Introduction to potential theory" , Acad. Press (1975) (Translated from German) MR0460666 Zbl 0188.17203 |
EncycloReader
is supported by the 
KSF