Catenary

The plane transcendental curve describing the form of a homogeneous flexible string of fixed length and with fixed ends attained under the action of gravity (see Fig.).

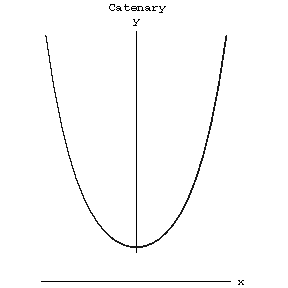
Figure: c020790a

In Cartesian coordinates its equation is $$y=\frac{a}{2}(e^{x/a}+e^{-x/a})=a\cosh \frac{x}{a}$$

The length of an arc beginning at the point $x=0$ is $$l=\frac{1}{2}(e^{x/a}-e^{-x/a})=a\sinh \frac{x}{a}$$

The radius of curvature is $$r=a{\cosh }^2\frac{x}{a}$$

The area bounded by an arc of the catenary, two of its ordinates and the $y$-axis is $$S=a\sqrt{y_2^2-a^2}-a\sqrt{y_1^2-a^2}=a^2(\sinh \frac{x_2}{a}-\sinh \frac{x_1}{a})$$

If an arc of a catenary is rotated around the $x$-axis, it forms a catenoid.

References[edit]