Continuous functions, space of
 From Encyclopedia of Mathematics - Reading time: 2 min
From Encyclopedia of Mathematics - Reading time: 2 min
A normed space  of bounded continuous functions
of bounded continuous functions  on a topological space
on a topological space  with the norm
with the norm  . Convergence of a sequence
. Convergence of a sequence  in
in  means uniform convergence. The space
means uniform convergence. The space  is a commutative Banach algebra with a unit element. If
is a commutative Banach algebra with a unit element. If  is compact, then every continuous function
is compact, then every continuous function  is bounded, consequently,
is bounded, consequently,  is the space of all continuous functions on
is the space of all continuous functions on  .
.
When 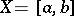 is a closed interval of real numbers,
is a closed interval of real numbers, 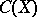 is denoted by
is denoted by  . According to the Weierstrass theorem on the approximation of continuous functions, the set
. According to the Weierstrass theorem on the approximation of continuous functions, the set  of all non-negative integral powers forms a complete system in
of all non-negative integral powers forms a complete system in 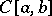 . (This means that the set of linear combinations of these powers, that is, polynomials, is everywhere-dense in
. (This means that the set of linear combinations of these powers, that is, polynomials, is everywhere-dense in 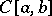 .) Consequently,
.) Consequently,  is separable; it also has a basis, for example, the Faber–Schauder system of functions forms a basis in
is separable; it also has a basis, for example, the Faber–Schauder system of functions forms a basis in  . A criterion for compactness in
. A criterion for compactness in  is given by the corresponding theorem of Arzelá: For a certain family of functions
is given by the corresponding theorem of Arzelá: For a certain family of functions 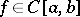 to be relatively compact in
to be relatively compact in  it is necessary and sufficient that the family is uniformly bounded and equicontinuous. This theorem generalizes to the case of the metric space
it is necessary and sufficient that the family is uniformly bounded and equicontinuous. This theorem generalizes to the case of the metric space  of continuous mappings from one metric compactum
of continuous mappings from one metric compactum 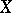 to another
to another 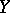 . For the compactness of a closed subset
. For the compactness of a closed subset  of
of 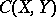 it is necessary and sufficient that the mappings in
it is necessary and sufficient that the mappings in  are equicontinuous. The distance between two mappings
are equicontinuous. The distance between two mappings 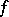 and
and  in
in 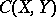 is given by
is given by
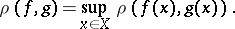 |
References[edit]
| [1] | P.S. Aleksandrov, "Einführung in die Mengenlehre und die allgemeine Topologie" , Deutsch. Verlag Wissenschaft. (1984) (Translated from Russian) |
| [2] | A.N. Kolmogorov, S.V. Fomin, "Elements of the theory of functions and functional analysis" , 1–2 , Graylock (1957–1961) (Translated from Russian) |
Comments[edit]
The Arzelá theorem is also known as the Ascoli–Arzelá theorem in the setting of functions on a compact metric space  . A sequence of functions
. A sequence of functions 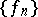 in
in 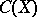 is relatively compact (i.e. the closure of the set
is relatively compact (i.e. the closure of the set 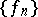 is compact), if the sequence is uniformly bounded (also called equibounded), i.e.
is compact), if the sequence is uniformly bounded (also called equibounded), i.e.  , and is equicontinuous (in
, and is equicontinuous (in  ), i.e.
), i.e.
 |
References[edit]
| [a1] | N. Bourbaki, "Elements of mathematics. General topology" , Addison-Wesley (1966) pp. Chapt. 10 (Translated from French) |
| [a2] | K. Yosida, "Functional analysis" , Springer (1978) pp. 68ff |
| [a3] | B.Z. Vulikh, "Introduction to functional analysis" , Pergamon (1963) |
17 views |
↧ Download this article as ZWI file
 KSF
KSF