Duality in complex analysis
 From Encyclopedia of Mathematics - Reading time: 8 min
From Encyclopedia of Mathematics - Reading time: 8 min
Let
The description of the dual spaces
Grothendieck–Köthe–Sebastião e Silva duality.[edit]
Let
Then one has the isomorphism (see [a1], [a2], [a3])
defined by
where
Duality and linear convexity.[edit]
When
A domain 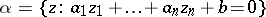 through
through
Some examples:
1) Let
2) Let
Let
If
where
Here,
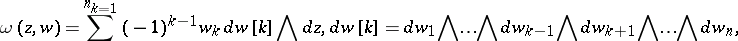 |
The index
A similar duality is valid for the space
A. Martineau has defined a strongly linearly convex domain
Duality based on regularized integration over the boundary.[edit]
L. Stout obtained the following result for bounded domains
is defined by the formula
Nacinovich–Shlapunov–Tarkhanov theorem.[edit]
Let
For any function
Here, the isomorphism (a3) can be defined by the formula (see [a14])
The integral is well-defined for some  for some
for some
Duality and cohomology.[edit]
Let
where
If
defined by the formula
where
A new result [a17] consists of the following: Let
where
Let
where
it is defined by the formula
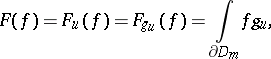 | (a7) |
where
Other descriptions of the spaces dual to spaces of holomorphic functions for special classes of domains can be found in [a18], [a19], [a20], [a21], [a22], [a23], [a24], [a10].
References[edit]
| [a1] | A. Grothendieck, "Sur certain espaces de fonctions holomorphes" J. Reine Angew. Math. , 192 (1953) pp. 35–64; 77–95 MR0062335 MR0058865 |
| [a2] | G. Köthe, "Dualität in der Funktionentheorie" J. Reine Angew. Math. , 191 (1953) pp. 30–39 MR0056824 Zbl 0050.33502 |
| [a3] | J. Sebastião e Silva, "Analytic functions in functional analysis" Portug. Math. , 9 (1950) pp. 1–130 |
| [a4] | A. Martineau, "Sur la topologies des espaces de fonctions holomorphes" Math. Ann. , 163 (1966) pp. 62–88 MR190697 |
| [a5] | L. Aizenberg, "The general form of a linear continuous functional in spaces of functions holomorphic in convex domains in |
| [a6] | L. Aizenberg, "Linear convexity in |
| [a7] | S.V. Zhamenskij, "A geometric criterion of strong linear convexity" Funct. Anal. Appl. , 13 (1979) pp. 224–225 |
| [a8] | M. Andersson, "Cauchy–Fantappié–Leray formulas with local sections and the inverse Fantappié transform" Bull. Soc. Math. France , 120 (1992) pp. 113–128 Zbl 0757.32008 |
| [a9] | S.G. Gindikin, G.M. Henkin, "Integral geometry for |
| [a10] | S.V. Znamenskij, "Strong linear convexity. I: Duality of spaces of holomorphic functions" Sib. Math. J. , 26 (1985) pp. 331–341 Zbl 0596.32017 |
| [a11] | S.V. Znamenskij, "Strong linear convexity. II: Existence of holomorphic solutions of linear systems of equations" Sib. Math. J. , 29 (1988) pp. 911–925 Zbl 0689.47004 Zbl 0672.47009 |
| [a12] | L. Aizenberg, S.G. Gindikin, "The general form of a linear continuous functional in spaces of holomorphic functions" Moskov. Oblast. Ped. Just. Uchen. Zap. , 87 (1964) pp. 7–15 (In Russian) MR0180699 |
| [a13] | E.L. Stout, "Harmonic duality, hyperfunctions and removable singularities" Izv. Akad. Nauk Ser. Mat. , 59 (1995) pp. 133–170 MR1481618 Zbl 0876.32003 |
| [a14] | M. Nacinovich, A. Shlapunov, N. Tarkhanov, "Duality in the spaces of solutions of elliptic systems" Ann. Scuola Norm. Sup. Pisa , 26 (1998) pp. 207–232 MR1631573 Zbl 0919.35040 |
| [a15] | J.P. Serre, "Une théorème de dualité" Comment. Math. Helvetici , 29 (1955) pp. 9–26 |
| [a16] | A. Martineau, "Sur les fonctionelles analytiques et la transformation de Fourier–Borel" J. Anal. Math. , 9 (1963) pp. 1–164 |
| [a17] | L. Aizenberg, "Duality in complex analysis" , Israel Math. Conf. Proc. , 11 (1997) pp. 27–35 MR1476701 Zbl 0907.46018 |
| [a18] | H.G. Tillman, "Randverteilungen analytischer funktionen und distributionen" Math. Z. , 59 (1953) pp. 61–83 Zbl 0051.08901 |
| [a19] | S. Rolewicz, "On spaces of holomorphic function" Studia Math. , 21 (1962) pp. 135–160 MR0154146 |
| [a20] | L. Aizenberg, B.S. Mityagin, "The spaces of functions analytic in multi-circular domains" Sib. Mat. Zh. , 1 (1960) pp. 153–170 (In Russian) MR124526 |
| [a21] | L. Aizenberg, "The spaces of functions analytic in |
| [a22] | L.J. Ronkin, "On general form of functionals in space of functions, analytic in semicircular domain" Soviet Math. Dokl. , 2 (1961) pp. 673–686 MR131577 |
| [a23] | S.G. Gindikin, "Analytic functions in tubular domains" Soviet Math. Dokl. , 3 (1962) |
| [a24] | S.D. Simonzhenkov, "Description of the conjugate space of functions that are holomorphic in the domain of a special type" Sib. Math. J. , 22 (1981) MR610784 |
33 views |
↧ Download this article as ZWI file
 KSF
KSF