Variety of groups
 From Encyclopedia of Mathematics - Reading time: 4 min
From Encyclopedia of Mathematics - Reading time: 4 min
A class of all groups satisfying a fixed system of identity relations, or laws,
where
varieties of groups form a complete modular, but not distributive, lattice. The product
Examples of varieties of groups: the variety
Let
The properties of a solvable variety of groups
where
A variety of groups is said to be a Cross variety if it is generated by a finite group. Cross varieties of groups are locally finite. A variety of groups is said to be a near Cross variety if it is not Cross, but each of its proper subvarieties is Cross. The solvable near Cross varieties are exhausted by the varieties
A variety of groups is said to be finitely based if it can be specified by a given finite number of identities. These include, for example, all Cross, nilpotent and metabelian varieties. It has been proved [8] that non-finitely based varieties of groups exist, and that the number of all varieties of groups has the power of the continuum. For examples of infinite independent systems of identities see [9]. A product of finitely-based varieties of groups is not necessarily finitely based; in particular,
A variety of groups is a variety of Lie type if it is generated by its torsion-free nilpotent groups. If, in addition, the factors of the lower central series of the free groups of the variety are torsion-free groups, then the variety is said to be of Magnus type. The class of varieties of Lie type does not coincide with that of Magnus type; each of them is closed with respect to the operation of multiplication of varieties [10]. Examples of varieties of Magnus type include the variety of all groups, the varieties
References[edit]
| [1] | H. Neumann, "Varieties of groups" , Springer (1967) |
| [2] | M.I. Kargapolov, V.A. Churkin, "On varieties of solvable groups" Algebra and Logic , 10 : 6 (1971) pp. 359–398 Algebra i Logika , 10 : 6 (1971) pp. 651–657 |
| [3] | J.R.J. Groves, "On varieties of solvable groups II" Bull. Austr. Math. Soc. , 7 : 3 (1972) pp. 437–441 |
| [4] | R.A. Bryce, "Metabelian groups and varieties" Philos. Trans. Roy. Soc. London Ser. A , 266 (1970) pp. 281–355 |
| [5] | W. Brisley, "Varieties of metabelian 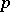 -groups of class -groups of class 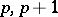 " J. Austr. Math. Soc. , 12 : 1 (1971) pp. 53–62 " J. Austr. Math. Soc. , 12 : 1 (1971) pp. 53–62 |
| [6] | A.Yu. Ol'shanskii, "Solvable just-non-Cross varieties of groups" Math. USSR Sb. , 14 : 1 (1971) pp. 115–129 Mat. Sb. , 85 : 1 (1971) pp. 115–131 |
| [7] | Yu.P. Razmyslov, "On Lie algebras satisfying the Engel condition" Algebra and Logic , 10 : 1 (1971) pp. 21–29 Algebra i Logika , 10 : 1 (1971) pp. 33–44 |
| [8] | A.Yu. Ol'shanskii, "On the problem of a finite basis of identities in groups" Math. USSR Izv. , 4 : 2 (1970) pp. 381–389 Izv. Akad. Nauk SSSR Ser. Mat. , 34 : 2 (1970) pp. 376–384 |
| [9] | S.I. Adyan, "The Burnside problem and identities in groups" , Springer (1979) (Translated from Russian) |
| [10] | A.L. Shmel'kin, "Wreath product of Lie algebras and their applications in the theory of groups" Proc. Moscow Math. Soc. , 29 (1973) pp. 239–252 Trudy Moskov. Mat. Obshch. , 29 (1973) pp. 247–260 |
| [11] | Yu.M. Gorchakov, "Commutator subgroups" Sib. Math. J. , 10 : 5 (1969) pp. 754–761 Sibirsk. Mat. Zh. , 10 : 5 (1969) pp. 1023–1033 |
Comments[edit]
The Oates–Powell theorem says that the variety generated by the finite groups is Cross. As a corollary it follows that the identities of finite groups admit a finite basis.
In [a1] the concept of varieties for a large class of algebraic structures was brought forward. The first systematic study of varieties of groups is [a2].
References[edit]
| [a1] | G. Birkhoff, "On the structure of abstract algebras" Proc. Cambridge Phil. Soc. , 31 (1935) pp. 433–454 |
| [a2] | B.H. Neumann, "Identical relations in groups I" Math. Ann. , 114 (1937) pp. 506–525 |
55 views |
↧ Download this article as ZWI file
 KSF
KSF