Wheel arrangement

Short description: Classification system for rolling stocks

Locomotives of different types (Whyte and UIC wheel notation)

In rail transport, a wheel arrangement or wheel configuration is a system of classifying the way in which wheels are distributed under a locomotive.^[1] Several notations exist to describe the wheel assemblies of a locomotive by type, position, and connections, with the adopted notations varying by country. Within a given country, different notations may also be employed for different kinds of locomotives, such as steam, electric, and diesel powered.

Especially in steam days, wheel arrangement was an important attribute of a locomotive because there were many different types of layout adopted, each wheel being optimised for a different use (often with only some being actually "driven"). Modern diesel and electric locomotives are much more uniform, usually with all axles driven.

Major notation schemes

The main notations are the Whyte notation (based on counting the wheels), the AAR wheel arrangement notation (based on counting either the axles or the bogies), and the UIC classification of locomotive axle arrangements (based on counting either the axles or the bogies).

Whyte notation is generally used for steam locomotives throughout the United States , Canada , the United Kingdom and Ireland. For diesels and electrics, North America uses the AAR wheel arrangement scheme while British practice uses a slightly simplified form of the European UIC classification scheme (except for small diesel shunters, where Whyte notation is used).

In mainland Europe, the UIC classification scheme is generally used for all locomotive types including steam, with some exceptions. In France , the UIC classification is used for diesels and electrics while a scheme similar to the Whyte notation, but counting axles instead of wheels, is used for steam locomotives. Notably, Switzerland had its own separate notation system until 1989, with the Swiss locomotive and railcar classification now only retained for its narrow gauge railways.

AAR wheel arrangement - Used largely throughout the US and Canada for diesel and electric locomotives.
UIC classification - Used in mainland Europe for all locomotive types. Used in the UK for electric and large diesel locomotives.
Whyte notation - Used in North America, the UK and Ireland for steam locomotives, and for shunter locomotives (US: switcher locomotives) in the UK.

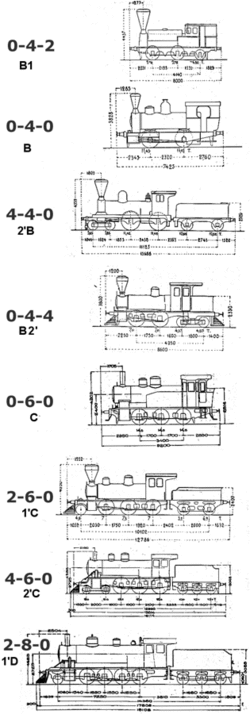
Comparison of wheel arrangements and wheel picture

VDEV/VMEV/UIC-system	Whyte-notation	American name	Picture scheme Locomotive front is to the left
A1	0-2-2		Oo
A2	0-2-4		Ooo
1A	2-2-0	Planet	oO
1A1	2-2-2		oOo
1A2	2-2-4		oOoo
2′A	4-2-0	Jervis	ooO
2′A1	4-2-2		ooOo
2A2	4-2-4		ooOoo
3A	6-2-0	Crampton	oooO
N/A	0-3-0		OOO
B	0-4-0	Four-Wheel-Switcher	OO
B1	0-4-2		OOo
B2′	0-4-4	Forney	OOoo
B3′	0-4-6		OOooo
1B	2-4-0	Porter	oOO
1′B1′	2-4-2	Columbia	oOOo
1B2′	2-4-4		oOOoo
1B3′	2-4-6		oOOooo
2′B	4-4-0	American, Eight-Wheeler	ooOO
2′B1′	4-4-2	Atlantic	ooOOo
2′B2′	4-4-4	Jubilee (CA), Reading (US)	ooOOoo
2′B3′	4-4-6		ooOOooo
C	0-6-0	Six-Wheel-Switcher	OOO
C1	0-6-2		OOOo
C2′	0-6-4		OOOoo
1′C	2-6-0	Mogul	oOOO
1′C1′	2-6-2	Prairie	oOOOo
1′C2′	2-6-4	Adriatic	oOOOoo
1′C3′	2-6-6		oOOOooo
2′C	4-6-0	Ten-Wheeler	ooOOO
2′C1′	4-6-2	Pacific	ooOOOo
2′C2′	4-6-4	Hudson, Baltic	ooOOOoo
D	0-8-0	Eight-Wheel-Switcher	OOOO
D1	0-8-2		OOOOo
D2′	0-8-4		OOOOoo
D3′	0-8-6		OOOOooo
1′D	2-8-0	Consolidation	oOOOO
1′D1′	2-8-2	Mikado	oOOOOo
1′D1′	2-8-2T	MacArthur	oOOOOo
1′D2′	2-8-4	Berkshire	oOOOOoo
1′D3′	2-8-6		oOOOOooo
2′D	4-8-0	Twelve-Wheeler	ooOOOO
2′D1′	4-8-2	Mountain, Mohawk (NYC)	ooOOOOo
2′D2′	4-8-4	Northern, General Service (SP), Golden State (SP), Niagara (NYC), Wyoming, Potomac (WM)	ooOOOOoo
2D3	4-8-6		ooOOOOooo
3′D3′	6-8-6	Turbine (Pennsylvania Railroad Steam Turbine)	oooOOOOooo
E	0-10-0	Ten-Wheel Switcher	OOOOO
E1′	0-10-2	Union	OOOOOo
1′E	2-10-0	Decapod	oOOOOO
2′E	4-10-0	Mastodon	ooOOOOO
1′E1′	2-10-2	Santa Fe	oOOOOOo
1′E2′	2-10-4	Texas, Selkirk (Canadian Pacific)	oOOOOOoo
2′E1′	4-10-2	Southern Pacific, Overland	ooOOOOOo
F	0-12-0	Pennsylvania, Twelve-Wheel-Switcher	OOOOOO
1′F	2-12-0	Centipede	oOOOOOO
1′F1′	2-12-2		oOOOOOOo
2′F1′	4-12-2	Union Pacific	ooOOOOOOo
2′G2′	4-14-4		ooOOOOOOOoo
Soviet (AA)	0-2-2-0		O O
B′B	0-4-4-0	nameless (Mallet)	OO OO
B′B1	0-4-4-2	nameless (Mallet)	OO OOo
2′BB2′	4-4-4-4	nameless (Pennsylvania Railroad Duplex)	ooOO OOoo
3′BB3′	6-4-4-6		oooOO OOooo
2′CB2′	4-6-4-4	nameless (Pennsylvania Railroad Duplex)	ooOOO OOoo
C′C	0-6-6-0	nameless (Mallet)	OOO OOO
(1′C)C	2-6-6-0	nameless (Mallet)	oOOO OOO
(1′C)C1′	2-6-6-2	nameless (Mallet)	oOOO OOOo
(1′C)C2′	2-6-6-4	nameless (Simple articulated)	oOOO OOOoo
(2′C)C2′	4-6-6-4	Challenger (Simple articulated)	ooOOO OOOoo
(1′C)C3′	2-6-6-6	Allegheny, Blue Ridge (Mallet/Simple articulated)	oOOO OOOooo
D′D	0-8-8-0	Angus (Mallet)	OOOO OOOO
(1′D)D	2-8-8-0	nameless (Mallet)	oOOOO OOOO
(1′D)D1′	2-8-8-2	nameless (Mallet)	oOOOO OOOOo
(1′D)D2′	2-8-8-4	Yellowstone (Mallet/Simple articulated)	oOOOO OOOOoo
(2′D)D1′	4-8-8-2	nameless (Simple articulated)	ooOOOO OOOOo
(2′D)D2′	4-8-8-4	Big Boy (Simple articulated)	ooOOOO OOOOoo
(1′E)E1′	2-10-10-2	Virginian (Mallet)	oOOOOO+OOOOOo
(1′D1′)(1′D1′)	2-8-2+2-8-2	Nameless, Garratt	oOOOOo+oOOOOo
(2′C1′)(1′C2′)	4-6-2+2-6-4	nameless (Garratt)	ooOOOo+oOOOoo
(2′C2′)(2′C2′)	4-6-4+4-6-4	nameless (Garratt)	ooOOOoo+ooOOOoo
(2′D)(D2′)	4-8-0+0-8-4	nameless (Garratt)	ooOOOO+OOOOoo
(2′D1′)(1′D2′)	4-8-2+2-8-4	Double Mountain (Garratt)	ooOOOOo+oOOOOoo
(2′D2′)(2′D2′)	4-8-4+4-8-4	nameless (Garratt)	ooOOOOoo+ooOOOOoo

Geared steam locomotives

Geared steam locomotives such as Shays, Heislers, and Climaxes do not have a standard wheel arrangement classification system. Instead of being classified by wheel arrangement, they are instead classified by their design and their number of trucks.

No. of trucks	American name	Picture scheme
2-truck	Class A Shay, Class B Shay, Class A Climax, Class B Climax, Heisler	oo oo
3-truck	Class C Shay, Class C Climax, Heisler	oo oo oo
4-truck	Class D Shay	oo oo oo oo