Probabilistic assessment of structures

This example deals with application of SBRA (Simulation Based Reliability Assessment) method for safety assessment of a welded steel beam with a sudden profile variation. Close attention is paid especially to the determination of the position where the profile changes.

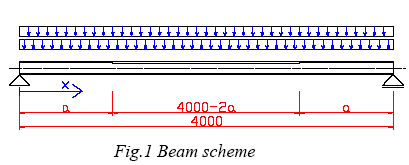
The beam is simply supported. Span is L = 4 m, material grade is steel S235. The beam is exposed to two uniformly distributed loads. The design values are: dead load g = 10 kN/m, long lasting live load q = 8 kN/m. Assess I-profiles of this steel welded beam (see Fig. 2) regarding the bending moment. Determine the position where the profile is to be changed with respect to the material savings. Lateral-torsion buckling of the beam is prevented. Do not assess the serviceability limit state. The design probability of failure is Pf,lim <= 0.00007.

The variable value of the load is equal to the product of its maximum value and the coefficient expressing its variation by the assumptive distribution, then g = 10 kN/m ∙ gvar, q = 8 kN/m ∙ qvar. Individual distributions follow in the chart (Fig.3).

The bending moment behavior along the beam Mx = ½ ∙ (g + q) ∙ (L ∙ x - x2) is traced by AntHill [2] software. The variables taking part in this simulation are g, q.

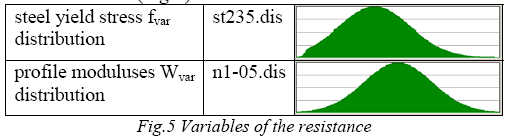
The variable value of the steel yield stress is equal to the coefficient expressing its variation by the assumptive distribution, then f = fvar. The variable values of the profile moduli are equal to the products of their nominal values and the coefficients expressing their variation by the assumptive distributions, then W1 = W1,nom ∙ Wvar, W2 = W2,nom ∙ Wvar. Individual distributions follow in the chart (Fig.5).

The nominal values of the cross-sections properties are W1,nom = 1.64E-4 m3, W2,nom = 1.25E-4 m3. The profiles 1 and 2 are displayed on Fig.2. The structure resistance is defined as the product Mr = W ∙ f, than Mr1 = W1 ∙ f, Mr2 = W2 ∙ f.

Critical cross-sections, considering the bending moment, are for Profile 1 the center of the beam (x = 2 m), for Profile 2 the place of its sudden profile variation (x = ?).

In the center of the beam's span is the failure probability Pf = 0.000053 < Pf,lim = 0.00007. Profile 1 satisfies the requirements, calculated by AntHill [1] software.

With assistance of AntHill [1] software, the probability of failures is found in individual positions near the estimated location of the sudden profile change.

The profile should be changed in position x = 1 m from the support. The failure probability in this location Pf = 0.000027 (see Fig.9) < Pf,lim = 0.00007. Profile 2 satisfies the requirements. Calculated by AntHill [1] software.

This study outlines the opportunity of using SBRA method for the global reliability assessment. The used example is very simple; however, it is possible to assess more complicated statically determined structures.

[1]Optimization Study of Beam with Sudden Profile Change; author Jan Hlavacek, Prague 2001

[2]Probabilistic Assessment of Structures using Monte Carlo Simulation – 1st edition; editors: P. Marek, J. Brozzetti, M. Gustar; publisher: ITAM CAS CR, Prague 2001