Unknown coordinate systems

At present, the geographic coordinates on Earth of latitude and longitude translate to right ascension and declination from the Greenwich meridian and the equator as projected on the celestial sphere.

But, other records may exist even here on Earth that use specific stars or bright lights in the sky especially at night that appear fixed relative to others.

This problem set is an attempt to acquaint you with alternative coordinate systems that use standard stars such as those in constellations to preserve a record of celestial events.

From your understanding of the unusual or unknown coordinate system try to determine the target star or the likely RA and Dec intended.

Angles between known RA and Dec for two stars or other objects may be found using NASA's Object Position Finder, Coordinate Converter, and Separation Calculator.

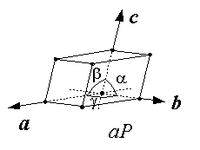
A triclinic coordinate system has coordinates of different lengths (a ≠ b ≠ c) along x, y, and z axes, respectively, with interaxial angles that are not 90°. The interaxial angles α, β, and γ vary such that (α ≠ β ≠ γ). These interaxial angles are α = y⋀z, β = z⋀x, and γ = x⋀y, where the symbol "⋀" means "angle between".

An observer has spotted an unknown object in the heavens. In the location of the observer and perhaps in time, the now common RA and Dec system is not available. In order to record this object, the observer constructs a device to indicate the objects position in the heavens relative to three well-known and easily seen stars. Once three rods are in place, each pointing to a specific star the observer marks off lengths on each rod until the combination of the three lengths locates the unknown object.

With the three lengths along each axis as a_x ~ 5, a_y ~ 6, and a_z ~ 8 marked, the observer measures the three interaxial angles at α = 26°, β = 82°, and γ = 64°.

The x-axis is pointing to Achernar. The y-axis is pointing to Rigel. And, the z-axis is pointing to Aldebaran.

Using pointers attached to each rod at ninety degrees swinging into the space between each rod, which star or stars are closest to the mutual point or points. What constellation(s) are they in. Use diagrams to justify your answer.

What is the RA Dec of the target?

In a monoclinic coordinate system, a ≠ b ≠ c, and depending on setting α = β = 90° ≠ γ, α = γ = 90° ≠ β, α = 90° ≠ β ≠ γ, or α = β ≠ γ ≠ 90°.

As in problem 1, an observer has spotted a luminous object and is attempting to fix its location in the sky using known stars.

The x-rod is pointing at Beta Librae (Zubeneschamali). The y-rod points at epsilon Ursa Major (eps UMa). And, the z-rod points at Altair. The angle between y and z is approximately 90°. Between z and x, β = 78°. Between x and z, γ = 76°. The angled pointers are 6 units along the x-axis and 8 units along the y and z-axes.

What constellation is the target object in?

What star or stars may be the target?

What is the RA Dec of the target?

An observer has located an object of interest using Betelgeuse, Arcturus, and Spica at equal units along each rod. What are the angles between the axes and the RA and Dec of the object?

An observer has spotted the Large Magellanic Cloud, find three stars to set up a coordinate system.

What are the angles between your three stars and the approximate units to pinpoint the LMC?

What is the RA Dec of your three stars?

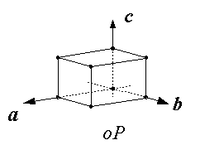
In an orthorhombic coordinate system α = β = γ = 90° and a ≠ b ≠ c.

An observer has located an object of interest at proportionately a = 6, b = 5, and c = 7 units along axes pointed at Altair, Alioth (epsilon Ursa Major, eps UMa), and Betelgeuse, where the angles between these stars are all approximately 90°.

What is the possible object of interest?

What are the errors on the angles between these stars?

What constellations are each star in?

What is the RA Dec of the target?

Locate three other bright stars that have interaxial angles of 90°.

A tetragonal coordinate system has α = β = γ = 90°, and a = b ≠ c.

Using Betelgeuse, Altair, an epsilon Ursa Major (Alioth), an observer has decided an object of interest has axes proportional units of 6 = a = b, c = 2.

What is the likely object and its RA and Dec?

What constellation is it in?

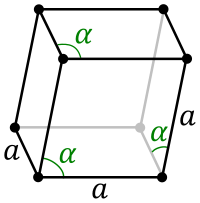
A rhombohedral system has a = b = c and α = β = γ < 120°, ≠ 90°.

Find three bright stars in which α = β = γ < 120°, ≠ 90°.

Find an object relative to these three stars where a = b = c, approximately.

A hexagonal system has a = b ≠ c and α = β = 90°, γ = 120°.

Find three stars where α = β = 90°, γ = 120°. For example, Polaris and Delta Orionis (Mintaka) are 90° apart.

Locate an object along these three rods where proportionately a = b ≠ c.

• International Astronomical Union
• NASA/IPAC Extragalactic Database - NED
• NASA's National Space Science Data Center
• Office of Scientific & Technical Information
• The SAO/NASA Astrophysics Data System
• Scirus for scientific information only advanced search
• SDSS Quick Look tool: SkyServer
• SIMBAD Astronomical Database
• SIMBAD Web interface, Harvard alternate
• Spacecraft Query at NASA.
• Universal coordinate converter

{{Mathematics resources}}

{{Radiation astronomy resources}}{{Reasoning resources}}