Inner Product
 From Conservapedia
From Conservapedia In linear algebra, an inner product 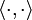 in a vector space
in a vector space 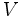 is a function from
is a function from 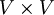 to
to 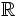 satisfying the following axioms for all vectors
satisfying the following axioms for all vectors  :[1]
:[1]
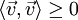 , with
, with 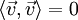 if and only if
if and only if  ,
,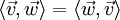 (the inner product is commutative),
(the inner product is commutative),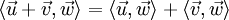 , and
, and- for all
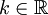 ,
, 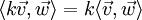 .
.
One consequence of the inner product axioms is that the inner product is multilinear in both variables; that is:
The dot product in the Euclidean vector space 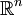 is the best-known example of an inner product.
is the best-known example of an inner product.
An inner product space is a vector space together with an inner product.
References[edit]
- ↑ Anton, Howard and Chris Rorres. Elementary Linear Algebra: Applications Version. 9th ed. N.p.:John Wiley & Sons, Inc., 2005. p. 296
Categories: [Mathematics]
↧ Download as ZWI file | Last modified: 02/23/2023 16:13:51 | 6 views
☰ Source: https://www.conservapedia.com/Inner_product | License: CC BY-SA 3.0
 ZWI signed:
ZWI signed:
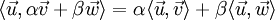
 KSF
KSF