Compton Scattering
 From Conservapedia
From Conservapedia Compton Scattering is the collision process between a X-ray or a gamma ray and a bound atomic electron where only part of the energy of the electromagnetic ray is transferred to the electron.
The effect was at first observed by Arthur Holly Compton in 1923 at Washington University in St. Louis and explained in his article "A Quantum Theory of the Scattering of X-ray by Light Elements".[1] Compton was rewarded the 1927 Nobel Prize in Physics for this discovery.
Arthur H. Compton treated the x-ray photons as particles and applied conservation of energy and conservation of momentum to the collision of a photon with a stationary electron..[2] He used the Planck relationship and the relativistic energy expression to derive the standard Compton formula:
Here, Δλ denotes the difference between the wavelengths of the incoming and the scattered ray, while θ is the angle of scattering.
Contents
The Compton Experiment[edit]
A collimated beam of high-energy photons, e.g. emitted from a radioactive 137Cs source or an X-ray emitter hits a target, e.g. a rod of graphite. A scintillation counter is used to measure the number and the energy of the deflected photons at various angles.
Classical Expectation[edit]
The number of photons should vary with the angle of the deflection, while the energy (i.e., the wavelength) of the photons should remain unchanged.
Observation[edit]
While indeed many of the scattered photons have an unchanged wavelength, there are some which lost energy, i.e., their wavelength lengthened. This lengthening depends on the angle of the deflection only.
Explanation[edit]
The lengthening of the wavelength happens when a photon interacts with a free electron in the material. The effect can be calculated along the following lines. The derivation requires relativity.
1) Conservation of energy: We assume that before the collision, the electron is nearly at rest, so its kinetic energy is zero:  . The photon has an energy of
. The photon has an energy of  , where
, where  is Planck's constant, and
is Planck's constant, and  is its initial frequency. After the interaction, the photon's frequency changed to
is its initial frequency. After the interaction, the photon's frequency changed to  , so its energy is now
, so its energy is now  . The kinetic energy of the electron after the collision is
. The kinetic energy of the electron after the collision is  . This is the relativistic energy of the electron minus its rest mass energy, given by E=m2. (Here,
. This is the relativistic energy of the electron minus its rest mass energy, given by E=m2. (Here,  is the mass of the electron.) We get the equation;
is the mass of the electron.) We get the equation;

(here,  is the momentum of the electron after the event)
is the momentum of the electron after the event)
2) Conversation of momentum: As we assume that the electron is nearly at rest at first, its initial momentum is zero. The momentum of the photon is at first  and then
and then  . The momentum of a photon can be calculated via
. The momentum of a photon can be calculated via  .
.
Looking at the picture, we see that

 , thus
, thus
I: 
Squaring  and rearranging leads to:
and rearranging leads to:
II: 
We equate our I and II and get:

This we rearrange to:

As for a photon  , we can bring this into Compton's form:
, we can bring this into Compton's form:

This describes exactly the effect observed by Compton! Compton himself used a similar derivation, also including the relativistic energy expression.
Probability of Compton Scattering[edit]
The probability for Compton scattering is approximately proportional to the atomic number Z, and for energies greater than 500 keV approximately proportional to  ,[3] the energy of the gamma ray photon.
,[3] the energy of the gamma ray photon.
References[edit]
- ↑ Arthur H. Compton: A Quantum Theory of the Scattering of X-ray by Light Elements, The Physical Review, Vol. 21, No. 5, May, 1923
- ↑ Compton Scattering Equation, Hyperphysics, C. R. Nave, Georgia State University
- ↑ Glossary of Nuclear Science Terms. Retrieved on January 10, 2013.
Categories: [Physics] [Physics Experiments]
↧ Download as ZWI file | Last modified: 02/14/2023 03:21:23 | 17 views
☰ Source: https://www.conservapedia.com/Compton_Scattering | License: CC BY-SA 3.0
 ZWI signed:
ZWI signed: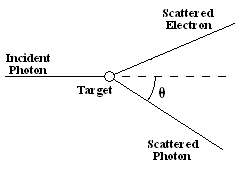
 KSF
KSF