Converge

From Conservapedia

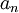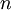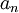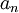In a metric space (X, d), a sequence $\text{[math]}$ in X is said to converge to a point $\text{[math]}$ if roughly speaking as $\text{[math]}$ goes to infinity $\text{[math]}$ gets closer and closer to $\text{[math]}$ and stays there. Rigorously, $\text{[math]}$ is said to converge to $\text{[math]}$ if for all $\text{[math]}$ there exists N such that for all n > N we have $\text{[math]}$ .

Similar definitions can be made for convergence of functions.

Categories: [Topology]

↧ Download as ZWI file | Last modified: 04/11/2024 16:14:17 | 28 views
☰ Source: https://www.conservapedia.com/Converge | License: CC BY-SA 3.0

✘

ZWI is not signed. [what is this?]