Vector Integration
 From Conservapedia
From Conservapedia Vector integration refers to four types of integrals of vectors:
- ordinary integrals, indefinite or definite
- line integrals: the vector values with respect to points on a line
- surface integrals: sum the vector values with respect to an area (performed as a double integral for each coordinate)
- volume integrals: sum the vector values with respect to a volume (performed as a triple integral for each coordinate)
Ordinary integrals[edit]
The ordinary (definite or indefinite) integral of a vector is done by integrating each orthogonal component separately.
Line integrals[edit]
The line integral of a vector is the summation of the dot product of the vector function with the position vector along the curve. In physics, an example of a line integral is the work performed by a vector force along an object as it moves along the line or path.
If the curve C is simple and closed (like a circle), then the value of the line integral is the "circulation" of the vector function about C, as in the case of a vector function that represents the velocity of a fluid.
The line integral of a "conservative vector field" around any closed curve is 0. The line integral of a conservative vector field from points P1 to P2 is independent of the curve chosen between those two points. If a vector function can be represented as the gradient of a single-valued, continuous function (as in the case of potential energy), then the vector function must be conservative and satisfy the above two conditions. The curl of such a vector function must then be zero.
Surface integrals[edit]
The surface integral of a vector is the summation of the dot product of the vector function with the outward unit normal perpendicular for each point on the surface. In physics this is known as the flux of the vector function over the surface region.
The surface integral of non-vector functions is also possible, as is the surface integral of the cross product of a vector function with the unit normal for every point on the surface.
Surface integrals are typically calculated by performing double integrals over two orthogonal coordinates.
Example[edit]
Calculate the surface integral of f(x,y) as the square root of x2 + y2 over an xy-planar region S bounded by x2 + y2 = 64:
Volume integrals[edit]
The volume integral of a vector or non-vector function is simply the triple integral of the function over the orthogonal coordinates of the space. It is useful in physics and mechanical engineering and plays a key role in the Divergence Theorem.
The volume integral is also known as the "space integral."
Categories: [Vector Analysis] [Calculus] [Mathematics]
↧ Download as ZWI file | Last modified: 02/26/2023 20:02:04 | 10 views
☰ Source: https://www.conservapedia.com/Vector_integration | License: CC BY-SA 3.0
 ZWI signed:
ZWI signed: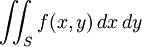
 KSF
KSF