Powers Of Ten
From Conservapedia Powers of ten are numbers of the form: 
where x is an arbitrary real number; they are an important concept in mathematics, science and economics.
Examples are 100 (x=2), 0.1 (x=-1), and 3.162 (x=0.5).
A list of positive, whole powers of ten:
| U.S. usage | Value | Former British usage |
|---|---|---|
| thousand | 103 = 1,000 | thousand |
| million | 106 = 1,000,000 | million |
| billion | 109 = 1,000,000,000 | thousand million or milliard |
| trillion | 1012 = 1,000,000,000,000 | billion |
| quadrillion | 1015 = 1,000,000,000,000,000 | thousand billion or (very rarely) billiard |
| quintillion | 1018 = 1,000,000,000,000,000,000 | trillion |
Logarithms[edit]
A logarithm is the inverse function of a power of ten:
If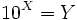 
then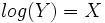 
For example: 
Logarithms are used in graphs using a logarithmic scale such as the Hertzsprung-Russell Diagram, these scales are mainly used to graph exponential functions that show an sharp increase beyond a specific value and would appear asymptotic on a conventional linear scale. Sometimes logarithms are also used to describe growth patterns in biology and economics.
Logarithms are not to be confused with the natural logarithm: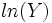 
which is based on Euler's number: e (approximately 2.71), instead of 10.
Scientific notation[edit]
Logarithms provide the basis for the scientific notation, which is used to write large numbers down without all of the zero's, instead the number is written as a number between 0 and 10 multiplied by a power of ten. When using scientific notation all numbers are rounded to two decimals by convention.
For example: 
or 
Economics[edit]
In economics powers of ten are important because they make the division of a currency into smaller units easier because multiplication by ten can be achieved by adding a zero. Until 1971 there were 20 shillings in an English Pound (£) and 12 pence in a shilling.[1] This made calculations difficult, that's why the Pound was decimalized in 1971. Today an English Pound is worth 100 pennies.
The first major currency to be decimalized was the Russian Rouble, in 1710.[2]
Decimalization of time[edit]
The ancient Egypt equivalent of a week was a ten-day period: a "decade".[3] Egyptians worked for eight days, the remaining days were free days.
In France [4] a decimal system was used to measure time between 1793 and 1805: 100 seconds made up on minute, 100 minutes made up one hour, 10 hours made up a day, 10 days made up a "décades", 3 décades made up a month and 12 months + five or six holidays made up a year. This system was very unpopular because it decreased the number of free days and undermined the Christian Sunday.
Today all time units smaller than a second (microsecond, nanosecond, etc.) are decimalized.
References[edit]
Categories: [Mathematics]
↧ Download as ZWI file | Last modified: 02/10/2023 00:53:05 | 10 views
☰ Source: https://www.conservapedia.com/Powers_of_ten | License: CC BY-SA 3.0
ZWI signed:
KSF