Solids
 From Conservapedia
From Conservapedia In calculus, solids are formed by rotating a curve around an axis and integrating to find the volume. Typically the integration is of slices cut vertically to the axis of the rotation that formed the solid. Those slices are then integrated from one end of the solid to the other.
Example[edit]
Consider the region in the first quadrant that has an upper bound of  and a lower bound of
and a lower bound of  , and bounded on the left side by the y-axis. Find the volume of the solid formed by rotating the region about the line
, and bounded on the left side by the y-axis. Find the volume of the solid formed by rotating the region about the line  .
.
Calculus provides an elegant way to determine the volume of this solid. First, find where the curves intersect in order to ascertain the end-point of the integration. The boundaries intersect where
or
This is best solved by trial-and-error. Since
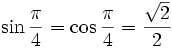 ,
,
that is the solution. The integral to find the volume of the solid must therefore be taken from x=0 on one side to:
on the other side.
We are now ready to find the volume. Note first that:
and thus
The next insight is to express r in terms of x. The variable r is the distance of the boundary from the axis about which it is rotated:
The volume then becomes:
The only challenging part of this integral is the last term, which must be integrated by parts:
Recall that:
and the solution to the overall integral is easy to obtain.
Categories: [Mathematics] [Calculus]
↧ Download as ZWI file | Last modified: 02/26/2023 15:00:34 | 23 views
☰ Source: https://www.conservapedia.com/Solids | License: CC BY-SA 3.0
 ZWI signed:
ZWI signed:






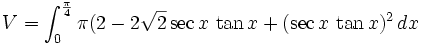


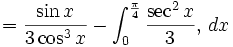

 KSF
KSF